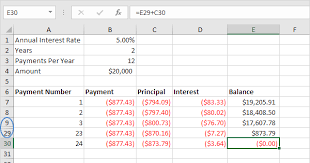
An Amortization Schedule
An amortization schedule refers to a tabular presentation of the mortgage loan payment schedule, indicating the interest and principal amount paid until the loan is repaid fully (Brechner & Bergeman, 2014). An amortization calculator is used in developing the schedule, based on the amount, interest rate and repayment period. The amortization schedule is generally utilized for identifying the amount paid, to both interest and principal, and the outstanding balance.
An amortization schedule helps in the generation of identical payment over the repayment period, such that the entire amount is paid by the end of the period (Biafore, 2013).
The schedule is used in determining the percentage of interest to be paid during each period in comparison to the principal amount to be repaid. In essence, it separates the portion of payment that covers the interest expense from the portion the premium paid to the principal in each period. Biafore (2013) notes that even though a similar amount of premium is paid towards the mortgage each period, the amount allocated to the principal and interest varies each time.
This variation can be observed from the amortization table. The amortization schedule ensures that the borrower and lender are on the same page with regards to the amount repaid and amount owed. This means that in case of any dispute, the schedule acts as reference on the history of payment and pending balances.
The amortization table is useful to the borrowers in that it is a basis for organizing their finances. The borrower is able to track payments made, interest paid and money owed at any given time; such that they can determine their home equity at any given time. Lenders on the other hand can track what is owed by borrowers.
Response 3
In amortizing the mortgage, a significant amount of the payments paid during the initial months mostly comprises of interest, while the remaining amount is paid to the principal (Biafore, 2013). The payments to interest then start declining as the mortgage is repaid, such that the interest paid in the later years is minimal or none.
Accordingly, the tax deducted based on home mortgage interest is likely to be higher during the initial years when a larger amount of interest is being paid, compared to later years when the interest being paid has reduced significantly. In this regard, it is logical to state that interest paid in earlier years plays a more helpful role in in tax reduction than interest paid in forthcoming years.
Response 4
An ordinary annuity differs from annuity due, mainly based on the timing. While the amount due in ordinary annuity is paid at each period end, an annuity due consists of cash flow series that occurs at each period’s beginning. The second difference is related with payment. In ordinary annuity, the payment done is associated with the period that precedes its date (Ehrhardt & Brigham, 2016).
Examples include mortgage payment, loans and coupon bearing bonds. Payment in an annuity due on the other hand, is associated with the period that follows its date. Examples include insurance premiums and rental lease payments.
Problems
- If interest rates are 8 percent, what is the future value of a $400 annuity payment over six years? Unless otherwise directed, assume annual compounding periods.
Future Value (FV) = P x [((1 + r) n – 1) / r]
Where P = Annual payments
r = Interest rate
n = Number of years
FV = 400 x [((1 + 0.08)6 -1)/ 0.08]
= $2,934.37
Recalculate the future value at 6 percent interest and 9 percent interest.
6% Interest
FV = 400 x [((1 + 0.06)6 -1)/ 0.06]
= $2,790.13
9% interest
FV = 400 x [((1 + 0.09)6 -1)/ 0.09]
= $3,009.33
- If interest rates are 5 percent, what is the present value of a $900 annuity payment over three years? Unless otherwise directed, assume annual compounding periods.
Present Value (PV) = P [(1 – (1 / (1 + r)n)) / r]
Where P = Annual payments
r = Interest rate
n = Number of years
PV = $900 [(1 – (1/(1+0.05)3))/0.05]
= $2,450.92
Recalculate the present value at 10 percent interest and 13 percent interest.
10 Percent
PV = $900 [(1 – (1/(1+0.1)3))/0.1]
= $2,238.17
13 Percent
PV = $900 [(1 – (1/(1+0.13)3))/0.13]
= $2,125.04
- What is the present value of a series of $1150 payments made every year for 14 years when the discount rate is 9 percent?
Present Value (PV) = P [(1 – (1 / (1 + r)n)) / r]
Where P = Annual payments
r = Interest rate
n = Number of years
PV = $1150 [(1 – (1/(1+0.09)14))/0.09]
= $ 8,954.07
Recalculate the present value using discount rate of 11 percent and 12 percent.
11 Percent
PV = $1150 [(1 – (1/(1+0.11)14))/ 0.11]
= $8029.15
12 Percent
PV = $1150 [(1 – (1/(1+0.12)14))/ 0.12]
= $7,622.39
References
Biafore, B. (2013). QuickBooks 2014: The Missing Manual: The Official Intuit Guide to QuickBooks 2014. Sebastopol, CA: O’Reilly Media, Inc.
Brechner, R. & Bergeman, G. (2014). Contemporary Mathematics for Business and Consumers, Brief Edition. Boston, MA: Cengage Learning.
Ehrhardt, M. C. & Brigham, E. F. (2016). Corporate Finance: A Focused Approach. Boston, MA: Cengage Learning.
Want help to write your Essay or Assignments? Click here